Many math textbooks move students very quickly into computation related to fractions...they start teaching them to find equivalent fractions by multiplying the numerator and denominator...having them "fill in the blank" to identify which fraction is greater, and so on. For students who learn computation easily, we sometimes miss when some other basic understandings are missing.
For this reason, I like to put my students in positions where they have to do a ton of "reasoning" and math talk to help ME learn about the level of their understanding--and for THEM to have to explain with precision their understanding. Check out this task we did to kick off our math class last week. I planned on 20 minutes--but we spent 45 VERY engaged minutes deepening our understanding!
Although we have been working on equivalent fractions recently, I have overheard a few discussions and comments from students that led me to believe that we may have a few misconceptions remaining about the idea of equal parts. We had already tackled a few--that you can't divide a circle by making vertical lines, you don't add denominators (working with "unit fractions" really helps with that misunderstanding!), and that equal parts don't all have to look the same. Check out THIS POST and THIS POST from last week to see more fraction lesson ideas.
On this day, I gave my students some "tricky" shapes and simply asked them with a team of three to decide if they were or were not divided into equal parts. The key? They had to be able to PROVE it so that others would understand their reasoning. They were told to use their piece of bulletin board paper to create a display to show their thinking.
For the first few minutes I got a ton of questions....
"Can we cut them out?" Sure.
"Can we draw on them?" Sure.
"Can we use glue?" Sure.
As they got to work, I circulated asking simple questions..."Are you sure?" and "Can you prove it?" and "Will others understand your explanation?". I heard some pretty faulty logic...things like
"It looks skinnier."
"It seems about the same size."
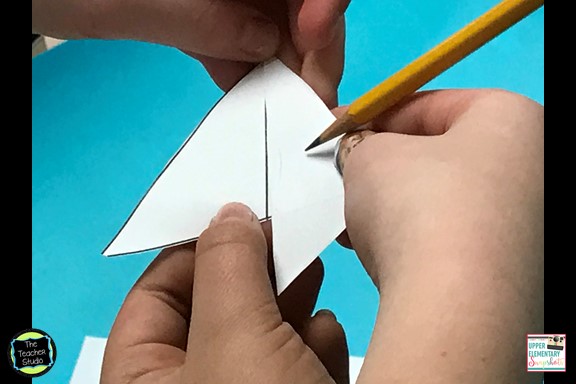
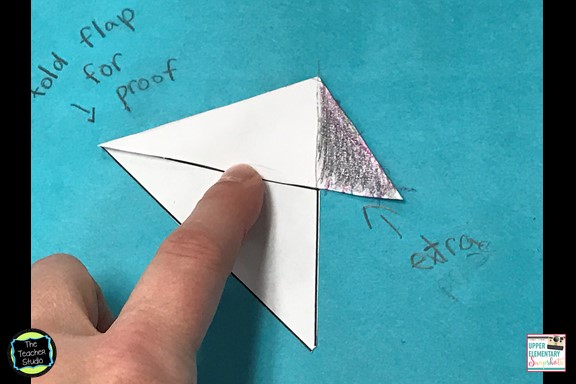
Each time, I probed the students to find a "mathematical way" to prove their ideas. When I passed this group, I noticed that they seemed to have a plan to prove the unequal parts of the triangle image. I asked them what they were doing...
They explained that when they folded the flap over, that they noticed "extra" so the edge pieces could not be equal to the middle section. They also admitted that at first they HAD thought it was divided into thirds because it "looked like it" so they were pretty excited to see that they had a mathematical explanation for something their eyes couldn't see.
Other groups were dismantling their shapes to use as templates to prove equal parts and had wonderful discussions about how pieces can be rotated in different directions and yet still be equal. Other groups started to use rulers to measure sides and claim that to be equivalent, the measurements needed to be the same. I quickly grabbed an index card and drew this:
I reminded the students that we had decided a week or so ago that this WAS, indeed, divided into equal parts because each piece was "a half of a half"--and then asked the team that was measuring if the measurements were the same. You could see their wheels turning...and some of them even started looking for ways to use the "half of a half" theory in their posters.One group was getting quite a bit of attention from the groups next to them for their organization. We've been talking about making our work "accessible" to others by organizing it, labeling it, and explaining clearly so this group got lots of compliments--and it motivated some other groups to "up their game" a bit!
After working for quite a while, we did a gallery walk where we checked out the work in the other groups, and then came up front. Different students offered to explain their thinking by using the document camera--and we practiced our accountable talk stems...agreeing, disagreeing, adding on, asking for clarification, and so on. We had a GREAT discussion and students used incredible thinking and math language to explain their ideas, to ask questions of each other, and to really force students to critique the thought processes and explanations of their classmates. Cool stuff!
So...I can't wait to continue our discussions as we look for other patterns and generalizations we can make as we move through our unit. This lesson is a version of one of the 16 lessons in my fractions unit--all geared toward really getting students to think deeply about fractions. Hope everyone is staying warm--and if you are teaching this next week like I am, may the force be with you!
Follow me on...
Instagram @Fourthgradestudio
Twitter @FourthGrStudio
Periscope @ FourthGrStudio